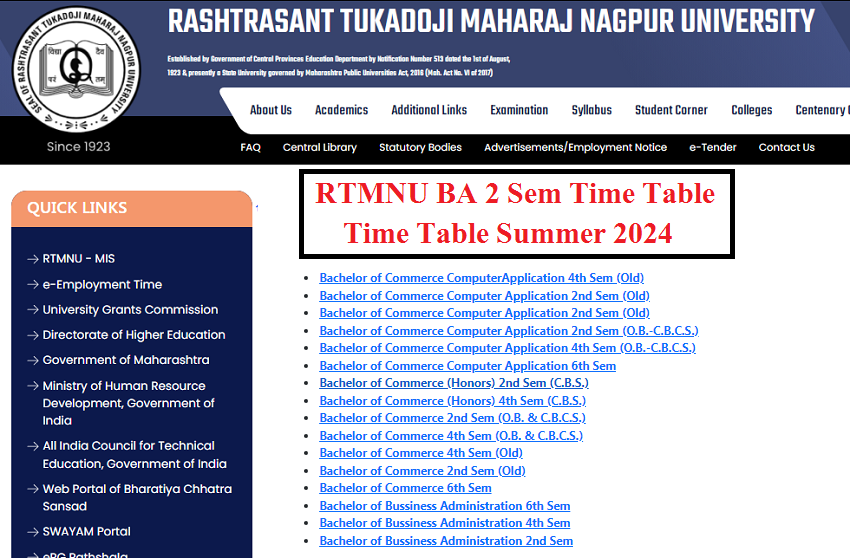
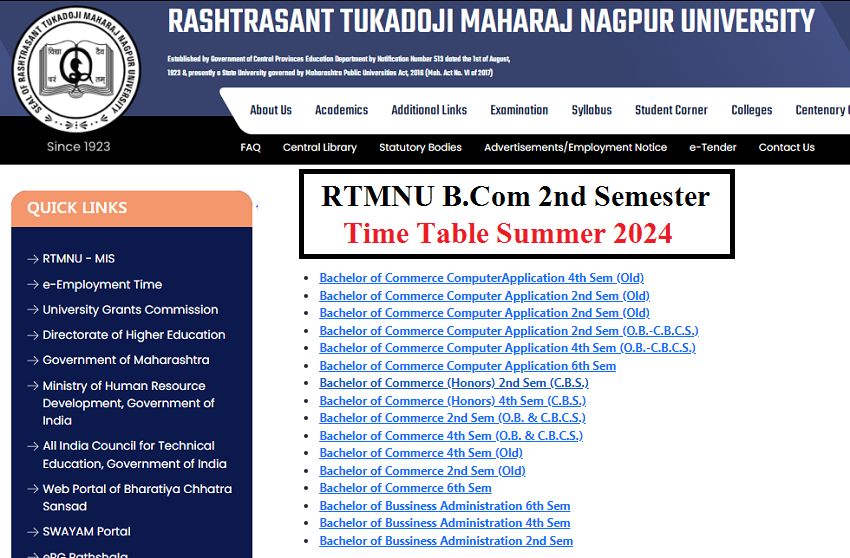
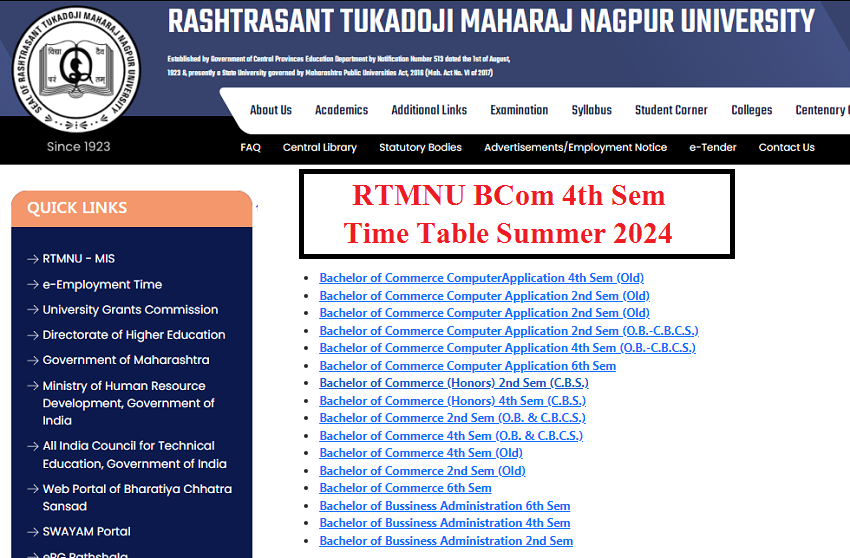
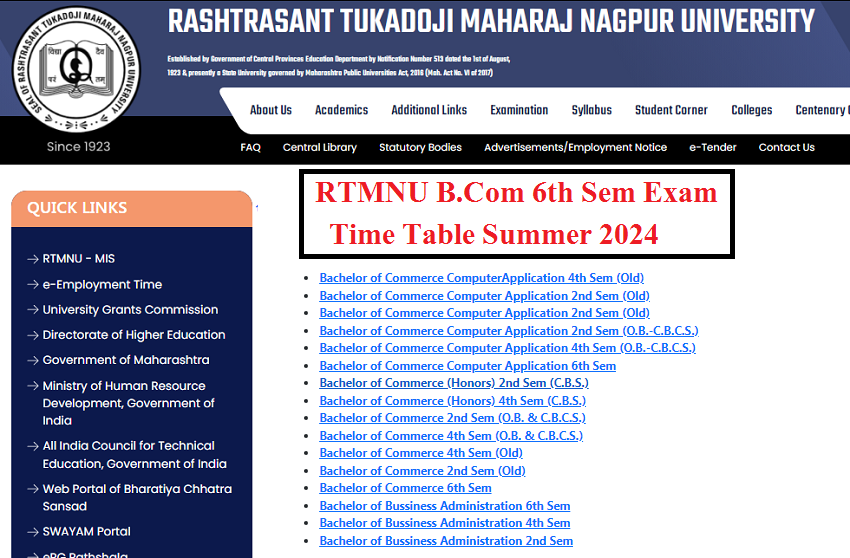
Savitribai Phule Pune University Syllabus for Ph.D Mathematics Course Work
Savitribai Phule Pune University
Syllabus for Ph.D. (Mathematics) Course Work
To be implemented from Academic year 2019 – 2020
(1) The Ph.D. program is a combination of Course work and Research work. The total
number of credits the student should get in the Ph.D. course work is 20.
(2) Course Work Structure :
PHD RM Research Mehodology 5
PHD OC Optional Courses(Student can take any TWO Optional Courses)10
PHD RG Reading course with the Research Guide(or an optional course in lieu of it) 5
Total Credits 20
(3) Only those candidates who have completed M.Phil. from any Statutory University and whose admission at M.Phil. was done through an Entrance Examination and Course work was prescribed at M.Phil. level, such candidates will be exempted from the Course work.
(4) If found necessary, course work may be carried out by candidates in sister Departments/Institutes either within or outside the University for which due credit will be given to them. The candidate can opt for such a course upon recommendation of the Guide and the Chairman, Ph.D. committee/HOD. Such course-work done outside the department should be restricted to 10 credit points.
(5) University Department research centre may introduce additional optional course (PHD OC) on recommendations of the Departmental Committee. The syllabus of the optional course will be prepared by the concerned teacher and will be flexible to accommodate new developments in that area. Whenever such an optional course is floated, the concerned syllabus will be discussed and approved in the Departmental Committee.
(6) The policies and procedures determined by the University will be followed for the conduct of examinations and declaration of the result of the candidate.
(7) The above mentioned courses with the given syllabi can also be considered as M. Phil. courses.
PHD RG : Research Methodology
(1) LATEX
(2) At least one Mathematical software out of the following: Scilab /KASH/ Maxima/ SAGE/Mathematica/Matlab. Other software suggested by the research guide
(3) Exposure to MathSciNet, JSTORE, Science-Direct, Scopus and other online journal database, writing review of a research paper
(4) Scientific writing .
(5) Plagiarism : awareness and usage of related softwares.
(6) Paper/ poster presentation in seminars/conferences/workshops.
PHD OC 01 : ATM School Participation
Advanced Training in Mathematics (ATM) school have been devised by National Board for Higher Mathematics to broaden the knowledge of a research student in Mathematics and also inculcating problem solving skills for better understanding of the subject and developing research orientation. A student participating in an NBHM sponsored ATM school of 3-4 weeks duration such as Annual Foundation School I for II or an Advanced Instructional School on a specific topic will earn 5 credits provided the school is attended after getting provisional admission for Ph.D. The Research Guide will request the coordinator of the school to give a grade to the student based on the participation of the student in the ATM school.
PHD OC 02 : Differential Equations and Dynamical Systems
(1) The Poincare return map: Local sections, Planar dynamics, Recurrence.
(2) Smooth Vector Fields: Differentiable functions, Differentiation in initial conditions, Linearization of nonlinear autnomus differential equations, Hamiltonian systems, Noether’s theorem.
(3) Hyperbolic Phenomenon: Hyperbolic linear vector fields, Perturbed Hyperbolic Systems, Contraction mapping principle, Local stable manifolds.
(4) Bifurcations: Implicit function theorem, Persistence of periodic points, Hopf bifurcations.
Reference Books:
1) N. G. Markley , Principles of Differential Equations, (Wiley Inter-Science 2004)
2) L. Perko, Differential Equations and Dynamical systems, Springer-Verlag (1991).
3) Hirsch and Smale, Differential Equations, Dynamical Systems, and Liner Algebra, Academic Press, New York, (1974).
PHD OC 03 : Advanced Partial Differential Equations
(1) Systems of PDEs, well-posed problems, classical solutions, weak solutions and regularity.
(2) Transport equation, Laplace equation, Heat equation and Wave equation.
(3) Nonlinear first order PDEs, complete integral, envelopes, charactristics,HamiltonJacobi equation, conservation law, shocks, entropy condition, Lax-Oleinik formula, weak solution, Riemann problem and Long time behavior.
(4) Similarity solutions, transform method, converting nonlinear in to linear PDE, Hopf-Cole transformation, potential functions, Hodograph and Legendre transforms, asymptotics, singular pertrubations, Laplace’s method, geometric optics, stationary phase, homogenization, power series, non-characteristics surfaces, real analytic functions and Cauchy-Kovalevskaya theorem
Reference Books:
• L. C. Evans, Partial Differential Equations, volume 19, American Mathematical Socity, 1998.
PHD OC 04 : Fractional Calculus and Fractional Differential Equations
(1) History of Fractional Calculus.
(2) Basic Definitions of fractional integral/derivative, Rimann-Liouville(RL) fractional integral/derivative, Caputo derivative, Gr¨unwald-Letnikov differ-integral, Composition rules, Lebniz rule for RL and Caputo derivative.
(3) Laplace Transform, Mittag Leffler functions and its variants, their properties. Integral representation of Mittag Leffler function and asymptotic expansions.
(4) Fractional Differential Equations (FDE):
(a) Linear FDE, transform methods for solving linear FDEs, such as Laplace. Fourier and Millin transforms.
(b) Nonlinear FDE, existence, uniqueness theorems, stability of solutions.
(c) Decomposition methods for solving nonlinear FDEs : Adomian method and New iterative method.
(d) Numerical Methods for solving FDEs such as fractional Adams method.
(5) System of linear FDEs : solution and properties.
(6) System of nonlinear FDEs : numerical solutions and study of related phenomena such as Chaos.
Reference Books:
• I. Podlubny; Fractional differential equations, Academic Press, San Diego, (1999).
• Oldham and Spanier, The Fractional Calculus : Theory and applications of
Differentiation and integration to orbitrary order, Dover, 1974.
• K. Diethelm; The analysis of fractional differential equations, Berlin: Springer,
(2010).
PHD OC 05 : Fourier Series and Functional Analysis
Conditional, unconditional and absolute convergence of a series in a normed linear space; notion of an orthonormal basis for a Hilbert space Trigonometric series, Fourier series, Fourier sine and consine series Piecewise continuous/smooth functions, absolutely continuous functions, functions of bounded variation (and their significance in the theory of Fourier series) Generalized Riemann-Lebesgue lemma Dirichlet and Fourier kernels Convergence of Fourier series Discussion (Without proofs) of some of the following topics: The Gibbs phenomenon, divergent Fourier series, term-by-term operations on Fourier series, various kinks of summability, Fejer theory, multivariable Fourier series.
Reference Books:
• Georage Bachman, Lawrence Narici and Edward Beakenstein, Fourier and Wavelet
Analysis, Springer-Verlag, New York, 2000
• Richard L. Wheeden and Antoni Zygmund, Measure and integral, Marcel Dekker
Inc., New York, 1977.
• Balmohan V. Limaye, Functional Analysis, New Age International (P) Ltd. New
Delhi, 2004.
PHD OC 06 : Commutative Algebra
Prime ideals and maximal ideals, Zariski topology, Nil and Jacobson, radicals, Localization of rings and modules, Noetherian rings, Hilbert Basis theorem, modules, primary decomposition, integral dependence, Noether normalization lemma, Krulls principal ideal theorem, Hilberts Null-stellensatz, Structure of artinian rings, Dedekind domains. Introduction to Algebraic Number Theory. Discriminants of number field.Factroisation of ideals. Finiteness of class number. Euclidean number rings.
Reference Book:
• Paul Ribenboim, Algebraic Number Theory
• M. F. Atiyah and I. G. Macdonald, Introduction to commutative algebra.
• P. Samuel, Algebraic Number Theory.
PHD OC 07 : Spectral Graph Theory:
Incidence matrix, path matrix, integer generalized inverse, Moore-Penrose inverse, adjacency matrix, eigenvalues of some graphs, trees, Laplacian matrix, Matrix-Tree theorem, non-singular trees, regular graphs, Perron-Frobenius theory, adjacency algebra of a regular graph, strongly regular graphs, eigenvalues of Caley graphs, Zeta functions on graphs(optional), the isoperimetric number for networks, expander graphs and examples of Ramanujan graphs.
Reference Book:
• R. B. Bapat, Graphs and Matrices, ( Hindustan Book Agency, Second Edition)
• Norman Biggs, Algebraic Graph Theory, (Cambridge University Press)
• Audrey Terras, Zeta Functions of Graphs, (Cambridge University Press)
• Series Sarnak Alain Davidoff Bruce Giuliana Valette Peter, Elementary Number
Theory, Group Theory and Ramanujan Graphs (Cambridge University Press)
PHD OC 08 : Ordered Algebraic Structures and applications to Graph Theory
(1) Partially ordered sets, Lattices, examples of lattices, Properties of lattices, Distributive and modular lattices. Boolean algebras
(2) Definition of the `-group, Basic facts, Absolute Values and the Triangle Inequalities in `-groups, `-permutations and `-homomorphisms, convex `-subgroups, ` -prime subgroups, values
(3) Definitions, examples, and basic properties, Some special `-rings, `-radical and `-prime `-ideals, Properties of maximal `-ideals, `-prime ideals, The hull-kernel topology on maximal `-ideals, minimal `-prime ideals
(4) Intersection Graphs, Cayley graphs and Zero-divisor Graphs: Intersection graphs of subgroups of groups and ideals of rings, Cayley graphs of groups, zero-divisor graphs of ordered sets, algebraic structures and inter-relationship between them.
Reference Book:
• M. R. Darnel, Theory of Lattice-Ordered Groups, Marcel Dekker, ISBN 0-8247- 9326-9.
• V. M. Kopytov and N. Ya. Medvedev, The Theory of Lattice-Ordered Groups, Springer, ISBN 978-90-481-4474-7.
• Stuart A. Steinberg, Lattice-ordered Rings and Modules, Springer ISBN 978-1- 4419-1720-1.
• Jingjing Ma, Lecture notes on the algebraic structure of lattice-ordered rings,World Scientific Research articles on intersection graphs and zero-divisor graphs of ordered structures and algebraic structures.Publishing Co. Ltd. ISBN 978-981- 4571-42-5
PHD OC 09 : Topics in Discrete Mathematics
Ihara zeta function, Ihara determinant formula, Covering graphs, Graph theory prime number theorem, Ihara zeta function of a weighted graph, Edge zeta functions, Definitions and Bass proof of the Ihara three-term determinant formula, Path zeta functions, Galois coverings of connected graphs, examples of coverings, Zigzag product of graphs, automorphism groups of some graphs, Chains in Distributive Lattices, The Incidence Algebra of a Locally Finite Poset, The Mobius Inversion Formula, Techniques for Computing Mobius Functions, Lattices and Their Mobius Algebras
Reference Book:
• Audrey Terras, Zeta Functions of Graphs, (Cambridge University Press).
• Audrey Terras, Fourier Analysis on Finite Groups and Applications, (Cambridge University Press, London Mathematical Society Texts-43).
• D. B. West, Introduction to Graph Theory, 2nd Edition (Pearson)
• Richard Stanley, Enumerative Combinatorics Vol 1 (Cambridge University Press, Second Edition).
• Krishnaiyan KT Thulasiraman, Subramanian Arumugam, Andreas Brandstdt,
Takao Nishizeki, Handbook of Graph Theory, Combinatorial Optimization, and
Algorithms,1st Edition (CRC Press)
PHD OC 10 : Algebraic Graph Theory
(1) Introduction: Graph theory, Linear algebra, Group theory.
(2) Eigenvalues of graphs: Some examples, Eigenvalues and walks, Eigenvalues and labllings of graphs, Lower bounds for the eigenvalues, Upper bounds for the Eigenvalues, Other matrices related to graphs, Cospectral graphs.
(3) Spectral graph theory, Star sets and star partitions, Star completes, Exceptional graphs, Reconstructing the characteristic polynomial, Non-complete extended p-sums of graphs, Integral graphs
(4) Graph Laplacians. The Laplacian of a graph, Laplace eigenvalues, Eigenvalues and vertex partitions of graph, The max-cut problem and semi-definite programming, Isoperimetric inequalities, The traveling salesman problem, Random walks on graphs.
(5) Auromorphisms of graphs: Graph auromorphisms, Algorithmic aspects, Automorphisms of typical graphs, permutation groups, Abstract groups, Cayley graphs, Vertex-transitive graphs.
(6) Distance-transitive graphs: Distance-transitivity, Graphs from groups, Combinatorial properties, imprimitivity, Bounds, Finite simple groups, The first step, The affine case, The simple scole case.
(7) Computing with graphs and groups: Permutation group algorithms, Strong and accessing a G-graph, Constructing G-graphs, graph, G-breadth-first search in a G-graph, Automorphism groups and graph is isomorphism, Computing with vertex-transitive graphs, Coset enumeration, Coset enumeration for symmetric graphs.
Reference Book:
• L. W. Beinke and R. J. Wilson,Topics in Algebraic Graph Theory, (Cambridge University Press).
PHD OC 11 : Complex Analysis
(1) Analytic functions, Path integrals, Winding number, Cauchy integral formula and consequences. Gap theorem, Isolated singularities, Residue theorem, Liouville theorem.
(2) Casorati- Weierstrass therorem, Bloch-Landau therorem, Picards therorems, Mobius transformations, Schwartz lemma, External metrics, Riemann mapping theorem, Argument principle, Rouches therorem..
(3) Runges theorem, Infinite products, Weierstrass p-function, Mittag-Leffler expansion.
Reference Books:
• Murali Rap and H. Stetkaer, Complex Analysis, World Scinetific, 1991.
• L. V. Ahlfors, Complex Analysis, McGraw-Hill, Inc., 1996.
• A. R. Shastri, Complex Analysis, 2010.
• S. G. Krantz, Complex Analysis: The Geometric View Points, Second edition, Carus Math. Monographs, MAA.
PHD RG : Reading course with the Research Guide
There will be a course conducted by the research guide of the Ph.D. student. As per the recommendation of the guide, the student may take a reading course with the guide or one of the optional courses in lieu of it.